
Forward Rate: Definition & Calculations
A forward rate is the future zero rate implied by today’s term structure of interest rates. Forward rates are interest rates for money to be borrowed between two dates in the future, but under terms agreed upon today.
Suppose R1 and R2 are known. If we invest $1 for 2 years it will grow to $(1+R2)^2 at the end of 2 years. Alternatively, one can invest $1 for one year, and make an arrangement for the proceeds for one additional year at the rate f. The growth will be $(1+R1)(1+f).
Therefore, if the market is free of arbitrage:
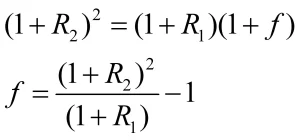
Formula for Forward Rates
Suppose that the zero rates for time periods T1 and T2 are R1 and R2 with both rates continuously compounded. The forward rate for the period between times T1 and T2 is:
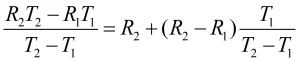
Forward Rate Agreement
A forward rate agreement is an agreement that a certain rate will apply to a certain principal during a certain future time period.
A forward rate agreement is equivalent to an agreement where interest at a predetermined rate, RK is exchanged for interest at the market rate. It can be valued by assuming that the forward interest rate is certain to be realized.
Consider a forward rate agreement between two parties X and Y for the time period T1 and T2.
RK: the rate of interest agreed in the forward rate agreement
RF: the forward LIBOR interest rate between time T1 and T2, calculated today
RM: the actual LIBOR interest rate observed in the market at time T1 for the period between T1 and T2
L: the principal underlying the contract
Cash flow to party X: L(RK−RM)(T2−T1)
Related Topics:
- What is a Forward Price? Definition & Formulas
- Financial Derivatives: Definitions and Types
- Futures Contracts – Overview & Basics
- Options Contract: Overview & Types
- Swaps – Definition & Types